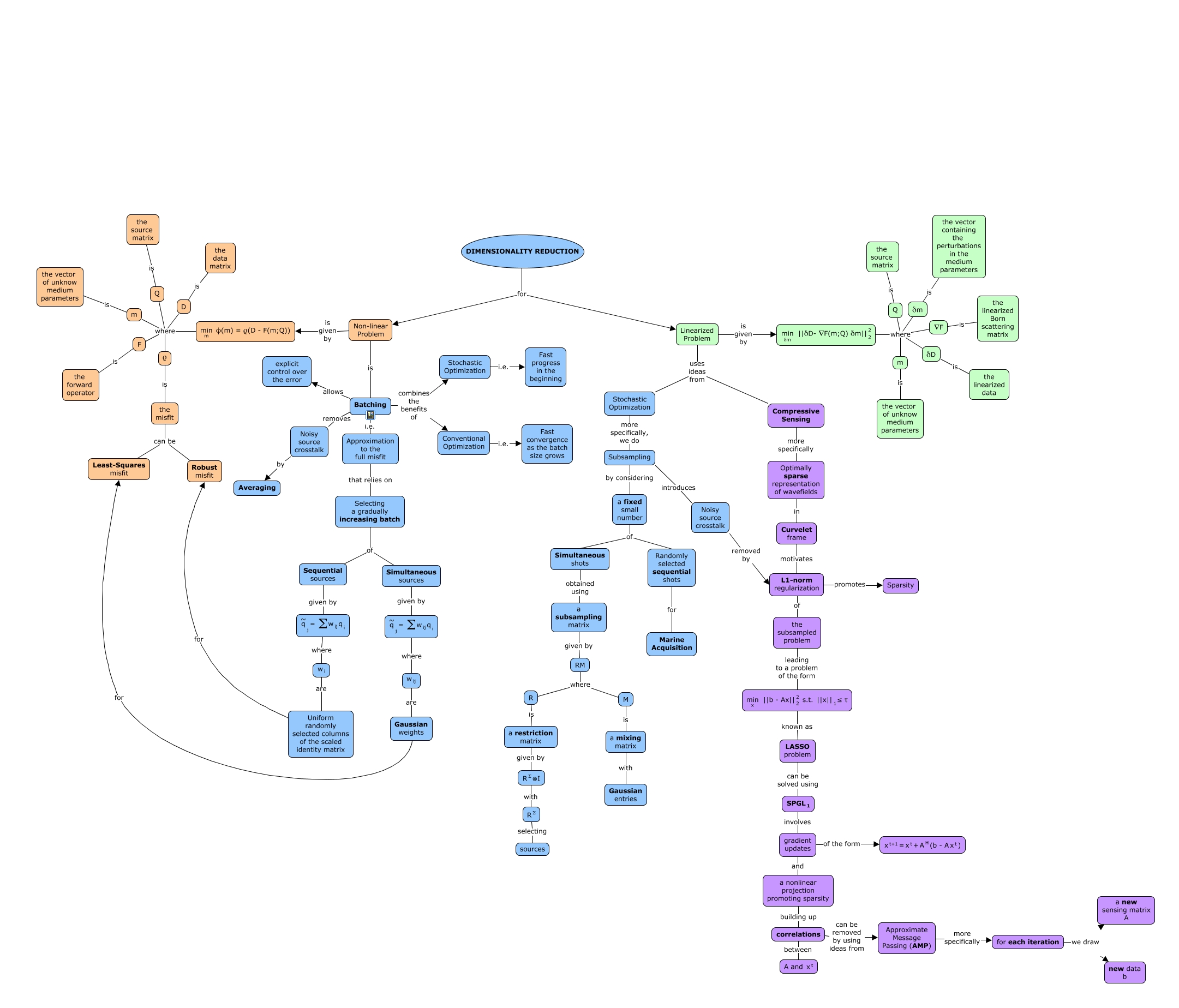
|
|||
|

|
|||
|
| A hybrid stochastic-deterministic optimization method for waveform inversion: Not Found |
| Hybrid Deterministic-Stochastic Methods for Data Fitting: Not Found |
| Robust inversion, dimensionality reduction, and randomized sampling: Not Found |
| Robust inversion via semistochastic dimensionality reduction: Not Found |
| Fast waveform inversion without source encoding: Not Found |